|
of a Fractal Nature photographic Math-Art essays highlighting mathematics in the natural world (geometric fractals mimic magnification/dilitational symmetry in Nature) inspired by the teachings and scientific investigations of Heinz-Otto Peitgen and Richard F. Voss |
|






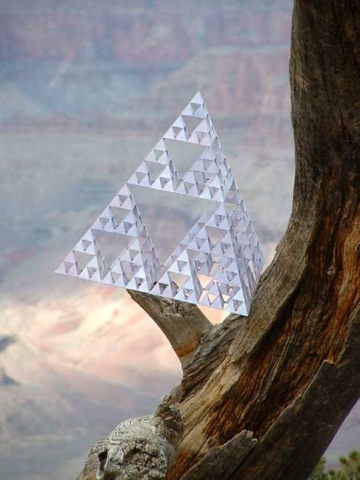



Shown here, the stage-4 Sierpinski tetrahedron provides a powerful visual introduction to fractal geometry and the concept of "self-similarity", in which a shape can be broken into smaller copies of the whole. Each new stage is composed of 4 smaller copies of the previous stage. As the number of stages increases, the Sierpinski tetrahedron approaches "exact self-similarity". |
Dodecahedron 
Dodecahedron Fractal Arizona Clouds and Sunsets Arizona Snowbowl thru-tetras: changing Aspen Atlantic Surf at Yamato (Boca Raton) Boyce Thompson Arboretum State Park Desert Botanical Gardens thru-tetras Butterflies at the Desert Botanical Gardens Everglades National Park Flagstaff in Fall Grand Canyon East Rim, Desert View Dr. Grand Canyon South Rim, Hermit's Rest Rte. Grand Canyon South Rim, Mather Pt. Gumbo Limbo Environmental Complex Morikami Museum and Japanese Gardens Oak Creek Canyon in Autumn Phoenix Zoo Red Rocks of Sedona Sedona viewed through tetras Plus: Butterfly Gallery (only butterflies) Boyce Thompson Arboretum in the Rain Duckweed (Lemna gibba) at The Phoenix Zoo First Images Math Structures in Falling Snow Various Mathematical Structures at ASU Windmills, Webs, and Lines (seen through tetras) |
|
|

